
As calculus developed further, infinitesimals were replaced by limits, which can be calculated using the standard real numbers.

This definition was not rigorously formalized.
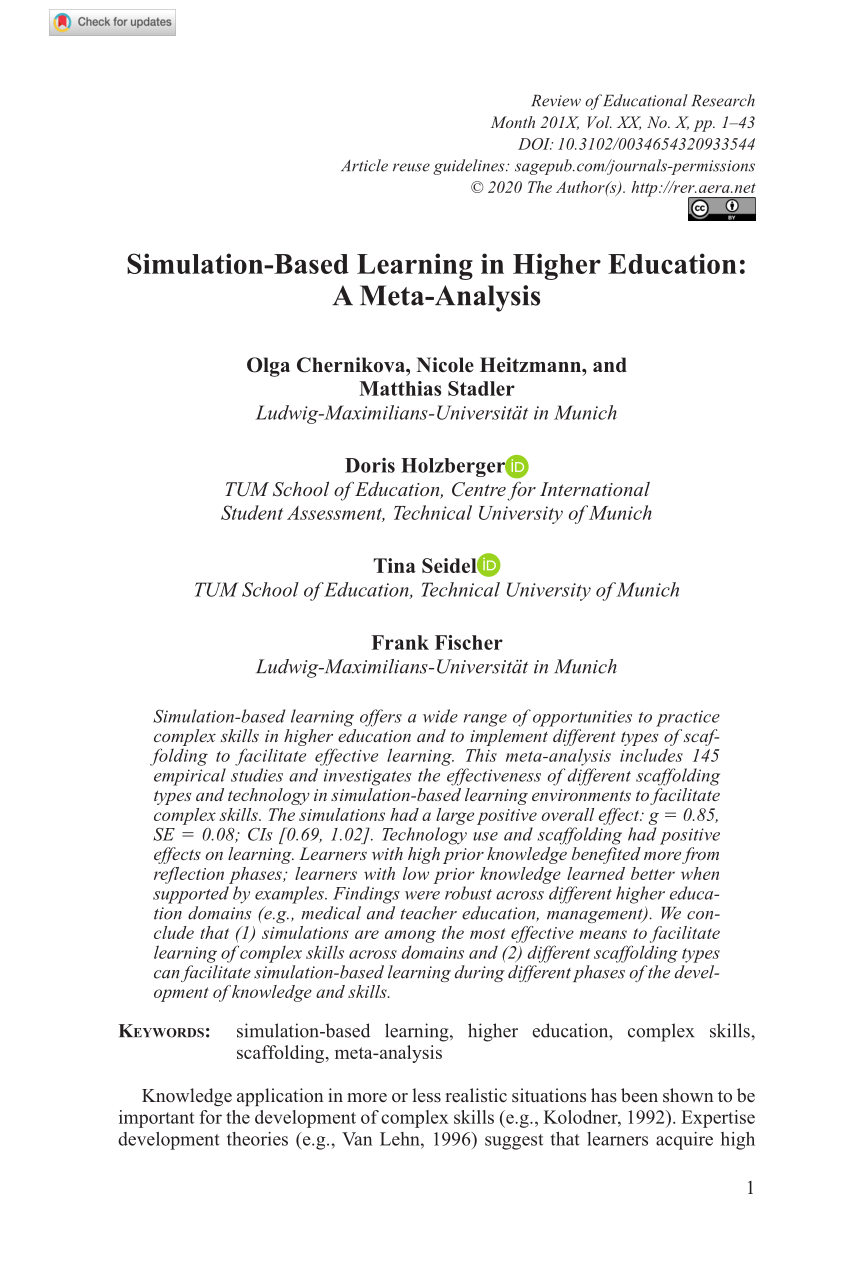
Infinitesimal numbers were introduced in the development of calculus, in which the derivative was first conceived as a ratio of two infinitesimal quantities. Infinitesimals do not exist in the standard real number system, but they do exist in other number systems, such as the surreal number system and the hyperreal number system, which can be thought of as the real numbers augmented with both infinitesimal and infinite quantities the augmentations are the reciprocals of one another.
#Continuity and infinitesimals pdf license#
This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( ).Extremely small quantity in calculus thing so small that there is no way to measure it Infinitesimals (ε) and infinities (ω) on the hyperreal number line (ε = 1/ω) Harmonic sierpinski gasket and applications. Primality, Fractality, and Image Analysis. The fractal nature of an approximate prime counting function. Mathematics of Bioinformatics: Theory, Methods and Applications John Wiley & Sons: New York, NY, USA, 2011 Volume 19. Spinger: Berlin/Heidelberg, Germany, 2005. Statistical physics for cosmic structures. The Fractal Geometry of Nature WH Freeman: New York, NY, USA, 1982 Volume 2. Trudy Matematicheskogo Instituta imeni VA Steklova 1951, 38, 176–189. Introduction to Mathematical Logic Chapman and Hall/CRC: London, UK, 2009. Frolov Centenary All-Russian Conference “Poet, Scientist, Teacher”, Syktyvkar, Russia, April 2009. A formalized language of complex hyperrational numbers theory. Complex hyperrational elementary functions. Modeling of basis aspects of real and complex analysis in the framework of hypernatural number axiomatic. Hyperrational numbers as the basis of analysis. Modeling the real analysis in the framework of axiomatic of hypernatural numbers. Inequality-Based Finite-Valued Predicate Logics Saint Petersburg University Press: Saint Petersburg, Russia, 2000. Thesis, Department of Mathematics and Statistics, Faculty of Science, University of Helsinki, Helsinki, Finland, May 2004. Constructive Nonstandard Analysis Without Actual Infinity. A Model for Intuitionistic Non-Standard Arithmetic. Constructive Proof Theory and Non-Standard Analysis URSS: Moscow, Russian, 2003. Über die Nicht-charakterisierbarkeit der Zahlenreihe mittels endlich oder abzählbar unendlich vieler Aussagen mit ausschliesslich Zahlenvariablen. Springer: Berlin/Heidelberg, Germany, 1981 Volume 891, pp. Lecture Notes in Mathematics Müller, G.H., Takeuti, G., Tugué, T., Eds. Internal set theory: A new approach to nonstandard analysis. Non-standard analysis with applications to economics. Nonstandard Methods in Stochastic Analysis and Mathematical Physics Academic Press: Orlando, FL, USA, 1986 Volume 122. Nonstandard Analysis for the Working Mathematician Springer: Dordrecht, The Netherlands, 2000. In Proceedings of the Conference in Modern Analysis and Probability, New Haven, CT, USA, 8–11 June 1982. A functional approach to nonstandard measure theory. Conversion from nonstandard to standard measure spaces and applications in probability theory. The Spectral Theorem-A Non-Standard Approach. Non-Standard Analysis Nord-Holland: Amsterdam, The Netherlands, 1966. The authors declare no conflict of interest. In fact, this allows us to approximate real numbers using hyperrational numbers, and shows a way to model real numbers and real functions using hyperrational numbers and functions. Also, we solve the task of line segment measurement using hyperrational numbers. We prove that a bounded monotonic sequence is a Cauchy sequence.


Elementary hyperrational analysis includes defining and evaluating such notions as continuity, differentiability and integral calculus. Since the theory of hyperrational numbers and axiomatic non-standard analysis is mainly published in Russian, in this article we give a brief review of its basic concepts and required results. In the axiomatic theory of non-standard arithmetic, hyperrational numbers are defined as triplets of hypernatural numbers. Unlike the non-standard model of arithmetic, this approach does not take models into account but uses an axiomatic research method. This arithmetic includes actual infinite numbers. This paper lies in the framework of axiomatic non-standard analysis based on the non-standard arithmetic axiomatic theory.


 0 kommentar(er)
0 kommentar(er)
